The Magic Mathworks Travelling Circus and Polydron
The children click two Polydron pieces together. They now have a hinge. When they realise with excitement that they can join a third piece to the two and move into three dimensions, their world expands.
For the past 26 years our touring maths lab, The Magic Mathworks Travelling Circus, has sought to bring to children a three-dimensional experience of mathematics. We lay out in a school hall suites of stations, each devoted to a particular topic according to the 'multiple embodiment' principle of Z. P. Dienes, (he of 'Dienes blocks'). One topic area the children gravitate to is 'Polyhedra'. Although we set particular tasks, what the children most like to do is build fantasy shapes. (We keep a voluminous photo album of the imaginative results.) When they stick to building the Platonic Solids, they love to nest one within another till, unbeknown to them, they have a model of the Cosmos as the Ancient Greeks conceived it. This picture is taken from a piece I wrote for Mathematics in School. It shows a sequence of Archimedean Solids. I move in reverse order, starting with a small solid and ending with an infinite polyhedron, a tiling:

Interesting things are always happening in 'Polyhedra'. I was working in the STFC labs at Daresbury shortly after Harry Kroto had won a Nobel prize for the discovery of fullerenes ('bucky balls') and new forms were constantly being discovered. A scientist rapidly clicked some Polydron pieces together and announced, "That was described in Nature last week".
On another occasion, a Y6 child had built a highly symmetrical solid which didn't conform to an Archimedean model. We noticed that it wobbled slightly, revealing that the face it stood on was not flat. Though mathematicians like perfect forms, chemists are used to such distortions. I wrote about them for Mathematics Teaching under the nonsense name 'Notquitohedra'. Here are 3 views of what, in honour of its creator, I call 'the Satterthwaite Solid':

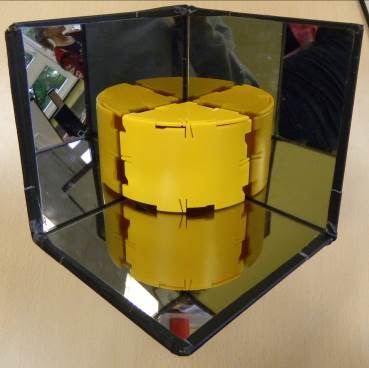
Polydron Sphera, the pieces with curved faces, presents a greater challenge, mentally and physically. I use a station which I think helps. At the centre is a 'mirror cube'. In it the children can drop, for example, ⅛ of a sphere. In the mirror they see the complete ball, revealing the symmetrical way the 8 necessary pieces are arranged. The children then build what they see. Here is a cylinder with end caps made from a ¼ disk and a ¼ cylinder:
The Frameworks pieces themselves can be used in quite challenging ways. The 4 standard colours lend themselves to colouring problems based on the theorem that no map (whether on the plane or the sphere) needs more than 4 colours. Here is a net of the Archimedean solid 34.5 map-coloured:

Though regular pentagons and decagons provide faces for polyhedra, they feature in no periodic tiling. However, they can if you overlap them. I had fun arranging Frameworks Decagons on the floor to make such a covering and put a piece in Mathematics in School, 'Tiles from coverings' ( PDF). Also listed is 'Notquitohedra' ( PDF) and an extract from the section 'Geomlab' on our website, 'Descartes' angle defect formula' ( PDF), where I've used Frameworks to show how Descartes may have arrived at the figure of two whole turns for the total of all the angular defects for a polyhedron. The angular defect is the amount by which the sum of the interior angles of the faces meeting at each vertex falls short of a whole turn. The piece I mentioned at the start was called 'The limiting case: sublime or ridiculous?' ( PDF). I've added it to the list. You may also like to look at the parts of our exhibition where Polydron features. ('Bestandsliste' is intended for German hosts, hence the spellings.) These are:
We also give workshops under the aegis of The Royal Institution, both in the U.K. and Germany. These are offered at Y6 and Y8+ level. In 'Polyhedra' we use Polydron Frameworks. Go to Masterclasses in hands-on mathematics and click on 'Polyhedra' for a PDF with notes for the advanced version.
Paul Stephenson, Magic Mathworks Travelling Circus