New Shapes for Old

Tandi Clausen-May uses three dimensional Polydron tiles to explore three dimensional shapes and their nets.

The ATM Easter Conference offers a host of different activities and experiences, but at the heart of them all is the opportunity to become immersed in mathematical problems, thoughts and explorations. These often arise from sessions or discussions at the conference itself, but last year I arrived with a problem already buzzing through my mind.

My question arose out of a mathematics enrichment day at a primary school, a week or so before the ATM Easter Conference. Josh and Len were using Polydron tiles to explore three-dimensional shapes and their nets. By clicking the tiles together and folding them up the boys discovered that they could make two (and only two) different shapes with eight triangular faces.

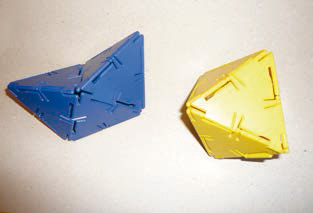
I admired the models, and we looked at their planes of symmetry. There were only two planes for the irregular octahedron, but, well, the regular one clearly had more.

“And of course”, I added casually, “they have different nets.”

“No they don’t,” said Len.

“Oh?” I said.

“You can unfold this one and then fold it back into that one”, said Josh.

“Show me”, I said.

So they cracked open their irregular octahedron and spread it out into a net.

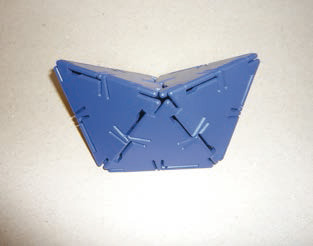
When they tried to fold it back into a regular octahedron, however, two of the faces overlapped.

“Is that the right net? Is it the one we used?” asked Len.

“No,” said Josh.

So the boys got back to work, and searched for their special net that would fold into two different octahedra. But, alas, the bell went for break and then the enrichment workshop moved on to a different activity. Research projects are like that, always being disrupted by administrative complications.

So, this is the problem I wanted to work on when I arrived at the ATM conference. Is there one net, made up of eight equilateral triangles, that will fold up to make two different octahedra?

I headed for the Workshop. The Workshop is at the very heart of the ATM Conference: a sort of Ala’a-Din’s Cave, packed with every sort of resource for the exploration of mathematics. From cubes and shapes and tiles through dozens of different kinds of paper and card, to tools such as scissors and string and glue, with endless puzzles and games. I made my way there, only to find that all the Polydron tiles had been taken for a session that was running later in the day. I wandered disconsolately back, drifted into the Publishers’ Exhibition, and found a whole table full of Polydron, overseen by Cyril Dowling, the Polydron Sales Director. He watched with somewhat bemused tolerance as I pushed part of his display out of my way, helped myself to eight triangles, and started the search.

It did not take long. The boys were right! Cyril seemed to be as delighted as I was and generously made me a present of the triangles.

There is a net that will fold up into two different octahedra. In fact, as I discovered later in the Workshop, there is more than one. But that, of course, just opens up more questions. We can find nets made with eight equilateral triangles that will fold up into different three-dimensional shapes. But what about a net made with more triangles? Or with fewer? Or with squares? Or pentagons? Or with a mixture of polygons? Or…

Now the hunt is on for nets that can offer new shapes for old. I wonder what we will discover at the next mathematics enrichment day!

Tandi Clausen-May is a freelance Mathematics Education consultant. She runs Able Maths enrichment days for Authors Abroad.