Kagome - The history of a pattern

In the case of our own planet the story begins around 4.3 billion years ago with the appearance of solid matter: ions joining to form crystals. We have to jump forward the same amount of time before organic life had evolved with the sophistication to discover their structures. Right up until the middle of the twentieth century this evidence was still indirect: we observed the scattering pattern when samples were bombarded by X-rays. But the electron microscope and its successors enabled us to identify individual ions. An important two-dimensional pattern discovered among minerals is the subject of this piece.

When did our ancient ancestors first use their hands to make things? What did they make? These questions cannot be answered because most materials break down with time so archaeologists and anthropologists usually only find hard objects like flint axe heads. But if we ask, what was the first thing we made which had a pattern, the most likely guess is: a basket. (A specimen found at Faiyum, upper Egypt, has been carbon-dated to at least 10,000 years B.P., well before the advent of pottery. Indeed, we know from their imprints on the finished object that baskets were often used to contain the clay for pots before firing.) As soon as we had the need to carry a large number of small objects, we had to make a material which was light, uniformly strong, didn't have large holes and was easy to make in quantity. Such mass production required the repetition of a simple process. Nature provides leaves. All you have to do is weave them together. If you alternate 'under' and 'over' weaves, you have strength, and this alternation immediately imposes a pattern. The simplest pattern is this:

Ours is the following, more sophisticated, pattern used by Japanese weavers - we don't know where it was first developed or how long ago:

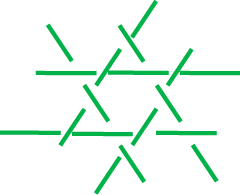
The weaving strands run in 3 directions mutually inclined at 120°.

Here is the pattern in plastic tiles:

This shows clearly the sets of parallel lines. They run across the picture, from bottom left to upper right, and from bottom right to upper left.

The tiling is uniform: taking a circuit of every vertex, we meet in order: triangle, hexagon, triangle, hexagon. Hence the notation 3.6.3.6. At every vertex a triangle meets a triangle and a hexagon meets a hexagon.

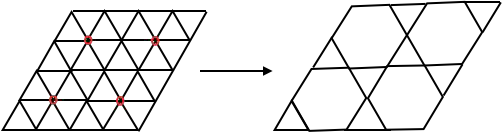
We can derive this tiling from 3.3.3.3.3.3 by removing the vertices of a rhombic tiling with twice the edge length of the original, together with the edges running from them:

The holes we produce are eyes ('me') in the basket ('kago'). Hence the name for this basketweaving style: 'kagome'.

If we are talking about crystals, we are interested in how this pattern appears as a cross-section of a three-dimensional structure. It results if we take a pack of cubes and section it like this:

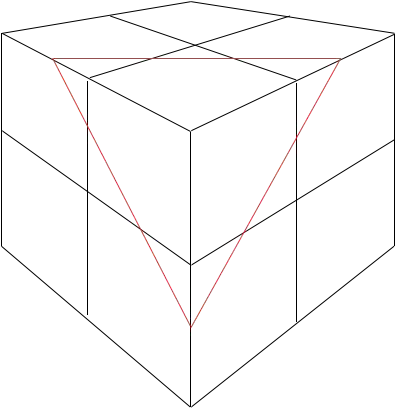
We can slice up the cube pack by 4 sets of parallel planes like that, each set parallel to one face of a regular tetrahedron, to make a packing of regular tetrahedra and truncated tetrahedra:

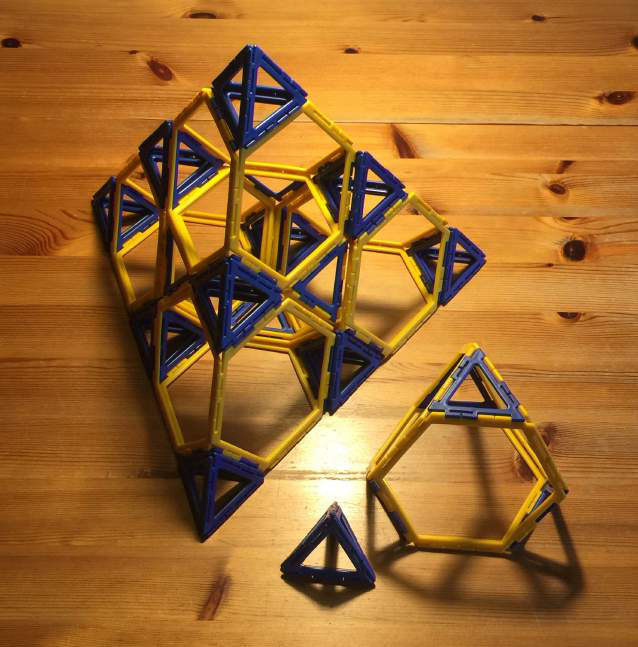
We now move right up to the present. For electrical engineers, the holy grail of materials science is to make something which is superconducting at room temperature. Potentially at least, we may now have it. An article in Nature for March 19, 2008, describes an alloy of iron and tin with alternate layers which have the kagome structure. The scientists were imitating, then, a structure already familiar to crystallographers. The layers are offset so that they look like this in plan view:

Where an 'up' triangle lies above a 'down' triangle, the vertices are joined by edges of the same length. The result is the shape below.

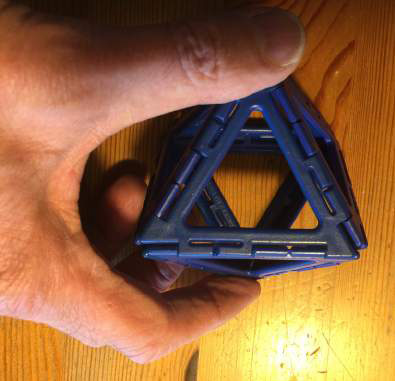
We could call this a triangular antiprism but, if we tilt it, as below, we realise that what we've got is simply a regular octahedron:

In 2000 George Lakoff and Rafael Núñez published a book called 'Where Mathematics Comes From' with the subtitle 'How the embodied mind brings mathematics into being'.

Mathematics is, among other things, the search for pattern. The basic rhythms of breathing and walking create a one-dimensional pattern: in the first case, one in time; in the second, one in both time and space. The fingers of the weavers create a one-dimensional spatial pattern which is more complex, a mathematical frieze. But, as they work with the parallel bamboo strands, they create - one might say are forced to create - a pattern in two dimensions. And the hands inform the brain. In the spirit of Rafael and Núñes I make the following conjecture:

1. The muscular sequences which produce the weave set up a mental template on which variants of the pattern can be built.
2. This leads to the mathematical concept of a periodic two-dimensional structure, a wallpaper pattern.

The phrase 'mental template' is a bit more than a metaphor. It represents a memory which is reinforced. A memory consists of a highly complex sequence of neuronal firings. The more often the initial memory is reinforced by contact with the same situation, the more likely that firing sequence is to remain active. Hence the slogan beloved of cognitive psychologists 'Use it or lose it'.

The move from 1 to 2 above instances the process of abstraction. We don't yet know how the brain achieves this. It seems to have a capacity for drawing analogies. We can see that this has survival value. We never meet exactly the same situation twice. Nevertheless, two situations are often sufficiently similar as to demand the same response. The brain, then, must have a mechanism for determining when two sets of sensory inputs are 'sufficiently similar'. The structure of an analogy is this:

A is to B in this context as X is to Y in that, in respect of such-and-such an attribute.

The mystery is that the 'attribute' does not announce itself: the brain must identify it. How it does this is a matter for on-going research. There's an irony here. Scientists are creating artificial systems with this capacity right now. But in order to do so they have so to program the systems that they cannot fully analyse what those systems are doing. As such systems take over more and more everyday tasks, the solution to this problem becomes increasingly urgent.

Paul Stephenson, Magic Mathworks Travelling Circus