Archimedes with Sphera

5 October 2016 | Paul Stephenson

Archimedes famously discovered that the volumes of a cone, a hemisphere and a cylinder of the same height and diameter stand in the ratio 1 : 2 : 3. He did this by hanging the solids on an imaginary balance and applying his ‘mechanical method’ (the law of the lever). The procedure yields geometry equivalent to this:

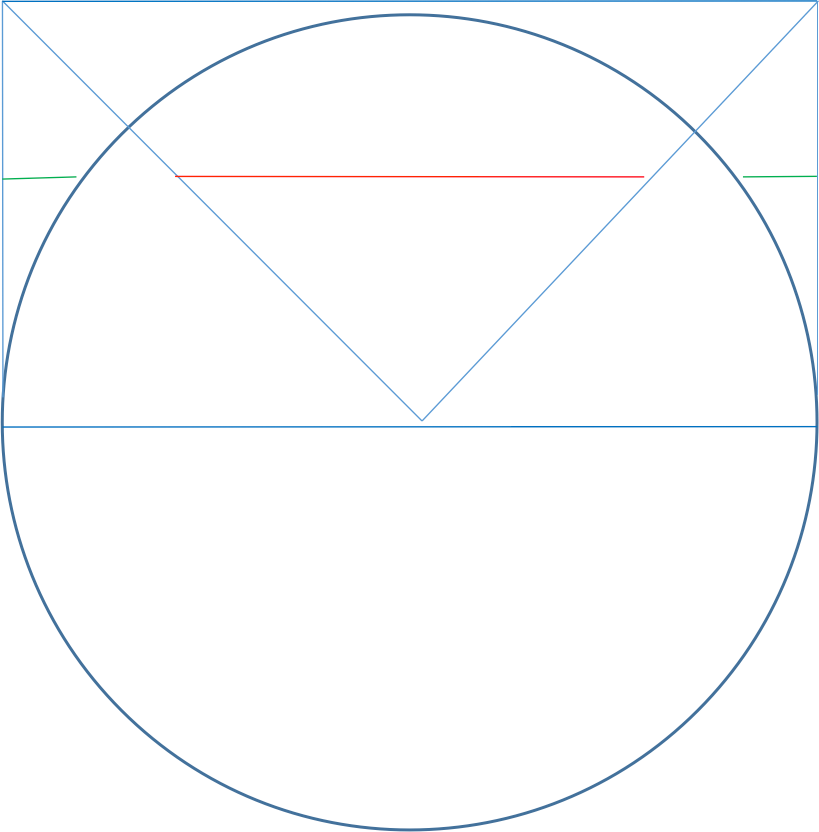
Using Pythagoras' Theorem, you can show that the red disk has the same area as the green annulus at the same height, and follow Archimedes in arguing that the equality holds as you build the solids up layer by layer ('3-D print' them if you like). You can confirm this empirically as follows:

Step 1 — **1. Shovel a pile of wet sand on to a board and compress with the hemisphere**

Step 2 — **2. Drop an open cylinder over the top**

Step 4 — **4. Tip the cone sand into the cylinder and pack down**

Step 5 — **5. The sand should fill the cylinder**

Paul Stephenson, Magic Mathworks Travelling Circus